

Are you ready to up your game and increase your chances of winning in online poker tournaments? Discover the secrets of poker hands with our comprehensive guide. From royal flushes to straights, we've got you covered. Learn the tricks, strategies, and expert insights to outplay your competition. Don't leave your success to chance; equip yourself with the knowledge that can turn the tables in your favour. Get ready to become a poker pro!
Dive into the thrilling world of poker with our guide to the best poker hands. Learn the strategies, rankings, and secrets behind these winning combinations. Whether you're a novice or a seasoned pro, this comprehensive resource will take your poker game to the next level. Join us in this exciting journey through the world of poker, and discover how the right hand can turn the tables in your favour. Get ready to shuffle the deck, make your bets, and chase that royal flush!
A Little Knowledge Is Always Dangerous! Educate Yourself About The Best Poker Hand Rankings And Create A Win-Win Situation. We at AceHigh Poker, strive for the betterment of our players by making concepts easier and fun to learn.
Winning and losing in poker depends majorly on what hand combinations you form with your dealt cards. If you are not good enough at different hand rankings, then you are risking your poker life as well as poker money. To be a good poker player and play any online card game, you must know what beats what.
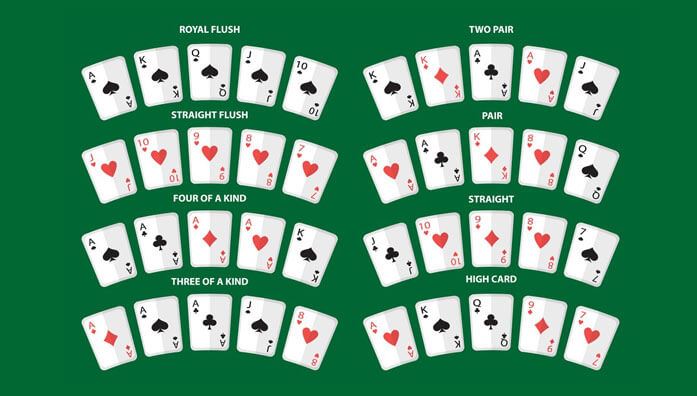
There are ten hands used to describe poker hand rankings. The highest being Royal Flush, is followed by a Straight Flush. “4 of a kind” is the third-highest hand. The next highest is a Full House followed by a Flush. A Straight is the next highest hand. The 4th lowest hand is “3 of a kind” followed by two pairs. The bottom two hands are one pair and the high card, the latter being the lowest in poker hand rankings.
So, Get Set, Go!

The highest among the best poker hands. It is a unique combination of an Ace, King, Queen, Jack and Ten each of the same suit. The probability of getting it is 0.000154% (odds as 649739:1). The Royal Flush is an invincible Hand because any player who possesses cannot be beaten under any circumstances.

The second-most difficult and the second-most invincible card combination, it features five suited cards in increasing numerical sequence. The probability of getting Straight Flush is 0.00139% (odds as 72192:1). Theoretically, the only way it can be beaten is by a Royal Flush.

The third in the list of priority of poker winning hands, it involves exactly four cards of the same rank plus one random card. It is the highest-ranking ‘Four of a Kind’ that is used to decide a winner. It has a probability of 0.0240% (odds as 4164:1) of occurrence.

It is a much simpler card configuration in which the cards are arranged in a 3+2 formation. It consists of three similar cards of one rank and another two similar cards of a different rank. A game changer, this Hand is used to decide the winner because the player with the highest ranking three cards is the winner. The probability of getting it is 0.1441% (odds as 693:1)

It consists of five cards of the same suit without any sequence. If several players get a Flush, then the winner is decided on the basis of card rankings. The probability of getting it is 0.1965% (odds as 508:1) among all poker winning hands.

It consists of five cards of different suits that are arranged in perfect numerical sequence. The highest-ranking card is used as the tie-breaker if such a case is found. A special case in it is that the Ace can act both as the High or Low card depending on the cards it is placed with. The probability of getting it is 0.3925% (odds as 254:1).

A relatively simple configuration that involves three cards of the same ranking and two random (unrelated) cards. This arrangement is used to decide in case of a tie-breaker. The probability of getting it is 2.1128% (odds as 46.3:1)

Two Pair Poker consists of two cards of the same rank, another two cards of some other rank that match each other but not the first pair, besides one card not of either rank.

It has two matched cards of the same rank, and three random and unrelated side cards. This is quite a common scenario to find it in every few online poker games in Texas Holdem hand rankings or those of Omaha as well.

It can be simply any card that does not fall into any of the categories mentioned above. It is best used to break the deadlock and determine the winner in case of a tie, provided none of the aforementioned hand rankings are available. If the first cards are identical, the second card is taken into account. If they are also identical, then the next one and so on until the first superior card is found.